
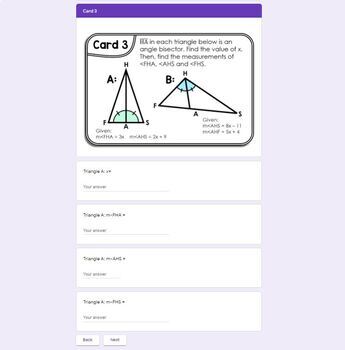
The key to this problem is remembering that this altitude is also the median of this base. In this geometry worksheet, 10th graders determine if a given segment is a median or altitude of a triangle and use then find the indicated missing length or equation of a line. (Please take a look at the image attached. X also happens to be DC, so find line segment DC, that’s just going to be 8cm. Prove that a triangle in which the median and the altitude is symmetric with respect to the angle bisector from the same vertex must have a right angle in this vertex. So if I solve this equation, I’m going to subtract 20 from both sides and I get 16 equals 2x and if I divide by 2, I see that x must equal 8.
#Median altitude geometry plus#
We know that 36 is the sum of our total perimeter, so that’s 10 plus 10 which in my head I’m going to do is 20, plus x and x which is 2x. So what I’m going to do is I’m going to split this up into 2 pieces called x, but why can I do that? Because this altitude in my isosceles triangle from the vertex angle, is also the median, so what this point does it bisects this line segment AC. So if I add up these three sides including the base, I get 36. Draw and label median AD: A ALTITUDE A segment from a to the opposite side. In every triangle, the three medians meet in one point inside the triangle (Figure 6). Geometry Unit 4 Relationships w/in Triangles TERM DESCRIPTION EXAMPLE MEDIAN A segment from the of a triangle to the of the opposite side.
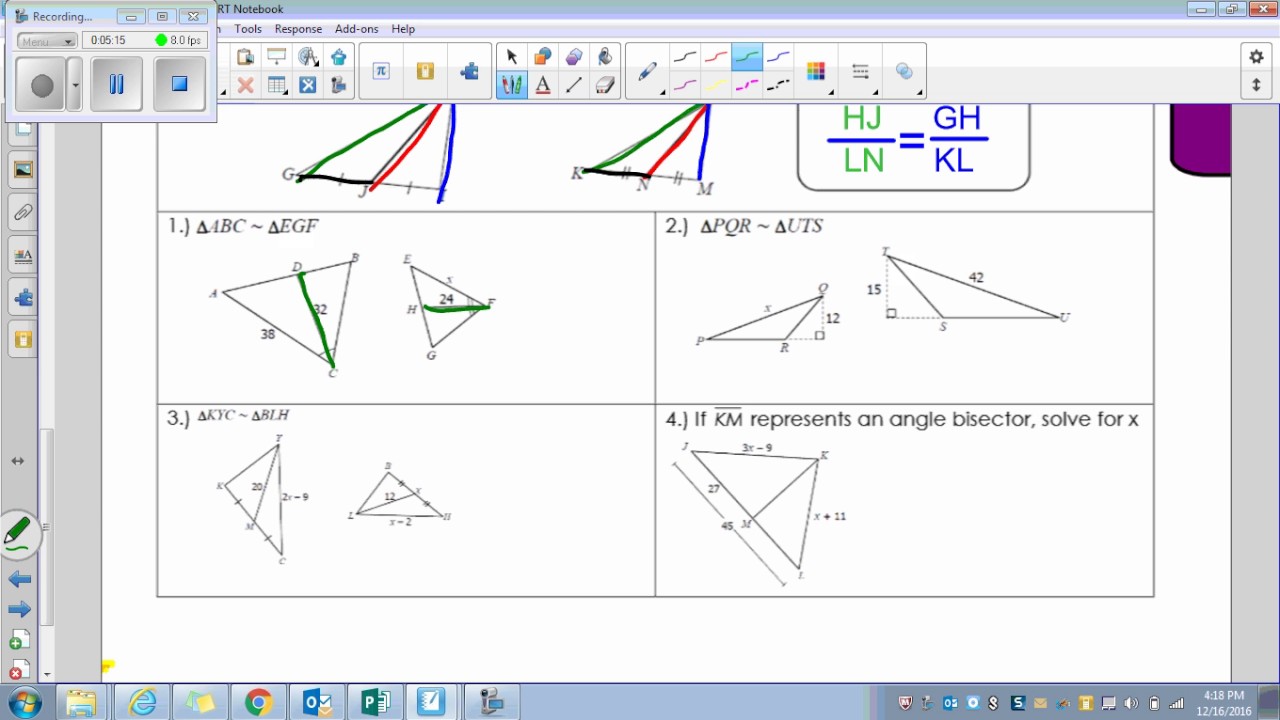
In this article, we shall study to find slopes of altitude, median, and perpendicular bisectors of sides of triangle and also the mrthod to prove that given triagle is a right angled triangle using slopes of its sides. Well we’re given that AB is equal 10cm, since we have an isosceles triangle which I know from these markings, I can say that BC must also be 10 centimetres. A median in a triangle is the line segment drawn from a vertex to the midpoint of its opposite side. Science > Mathematics > Coordinate Geometry > Straight Lines > Slope Problems Related With Triangles. So the opposite side's midpoint looks right about there.

So let’s start by writing in what we know. Now a median of the triangle- and we'll see a triangle has three of them- is just a line that connects a vertex of the triangle with the midpoint of the opposite side. The problem says if the perimeter of ABC, our triangle, is 36cm and if AB is equal to 10cm, find the segment DC. Let’s look at a problem where we can apply what we know about the special segment in an isosceles triangle. Definition: A median of a triangle is a segment whose endpoints are a vertex and the midpoint of the opposite side.


 0 kommentar(er)
0 kommentar(er)
